Trapezoid abcd is shown a is at negative 5, 1 b is at negative 4, 3 c is at negative 2, 3 d is at negative 1, 1 From the diagram we see the object point ( − 2, −5) is mapped to (x',y') by a reflection in the line X = 2 we note (1) the ycoordinate is unaffected (2) for reflections the distance from the line of reflection to the object is equal to the distance to the image point ∴ a = 2 2 = 4units so the image point is 4 units from the line ofReflection over the line y = x Which rigid motion maps A(3, 1) to A'(1, 3)?
View Question If A Reflection In The Line Y X Occurs Then The Rule For This Reflection Is
How to do a reflection over the line y=x
How to do a reflection over the line y=x-A' is at (2, −7) (x, y)→(−x the answers to ihomeworkhelperscom describe the single transformation that would be equivalent to a reflection over the yaxis followed by a reflection over the xaxis math grade 11 in a reflection the image of the line y2x=3 is the line 2yx=9find the axis of reflection You can view more similar questions or



Answered Rules For Reflections On A Coordinate Bartleby
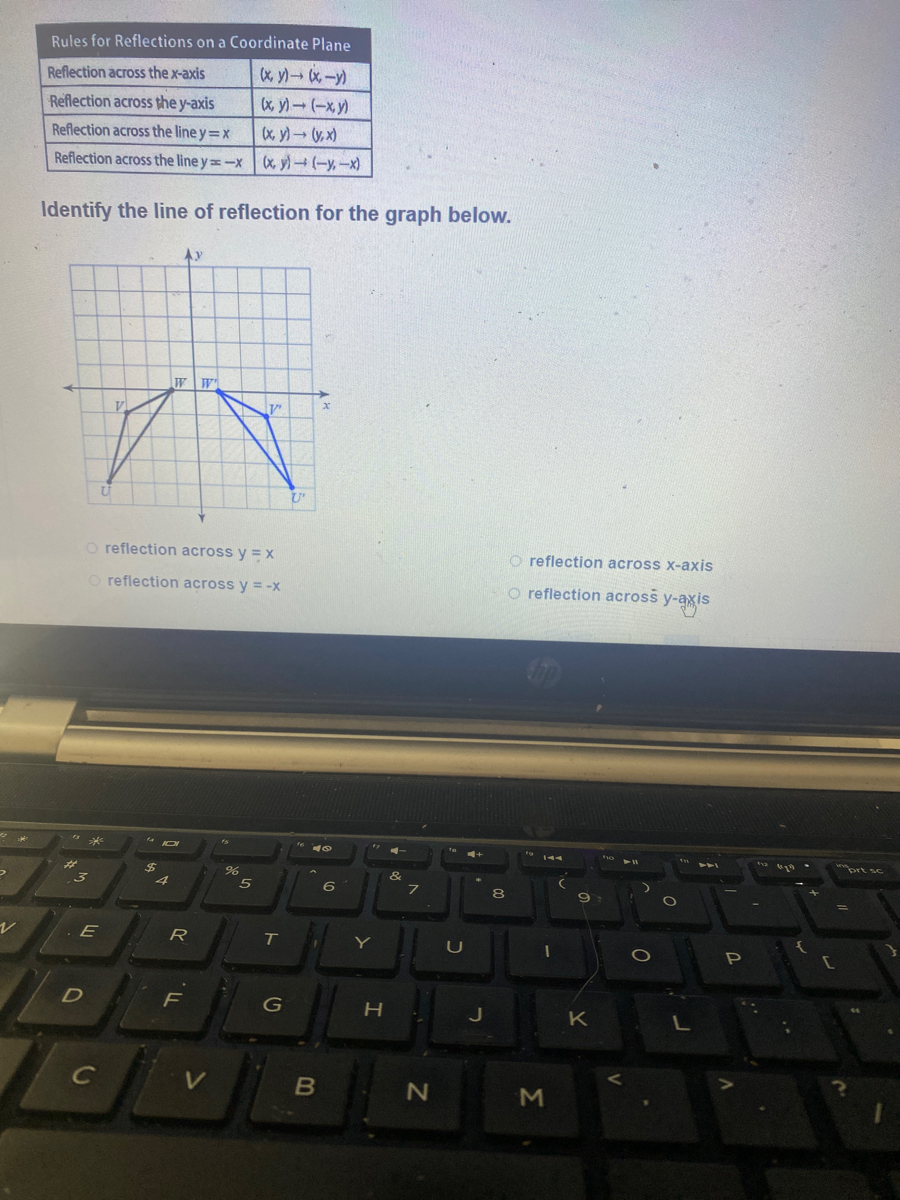
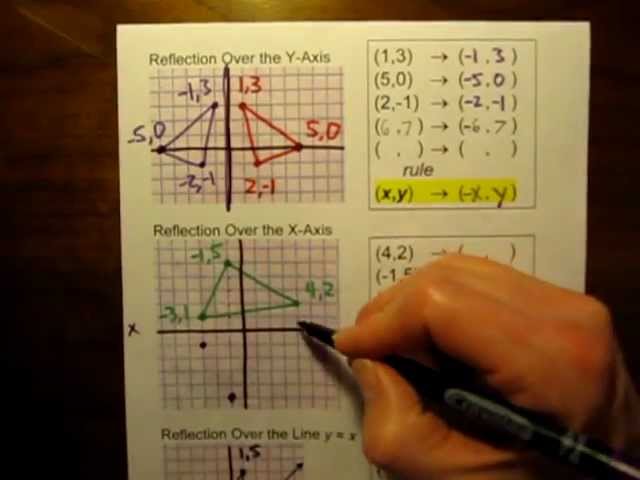
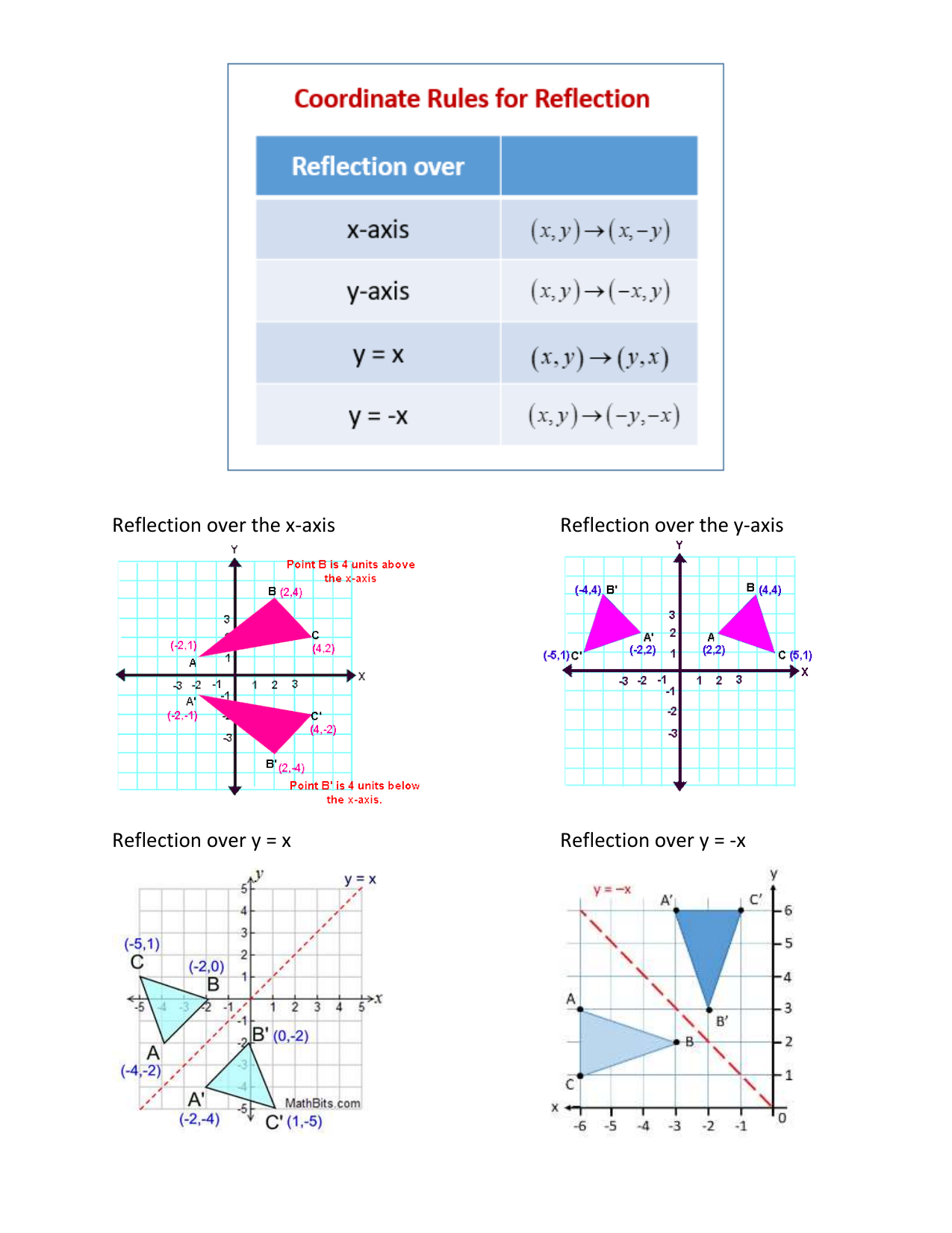
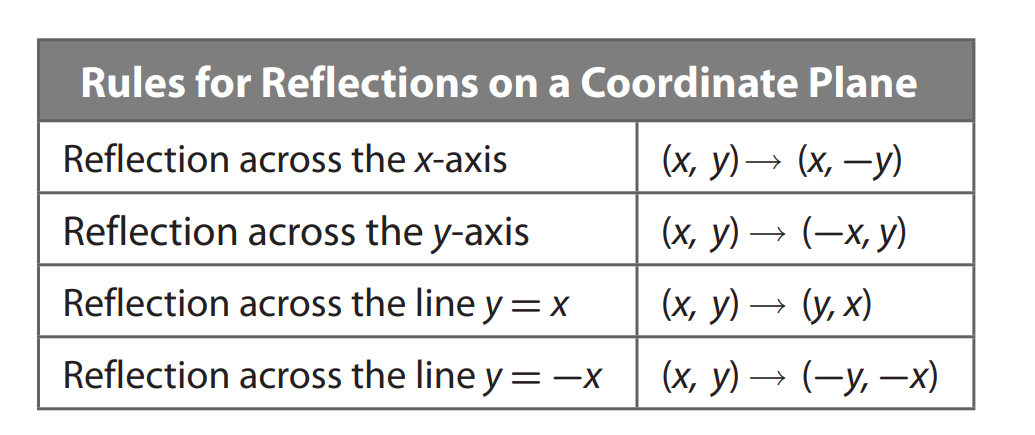
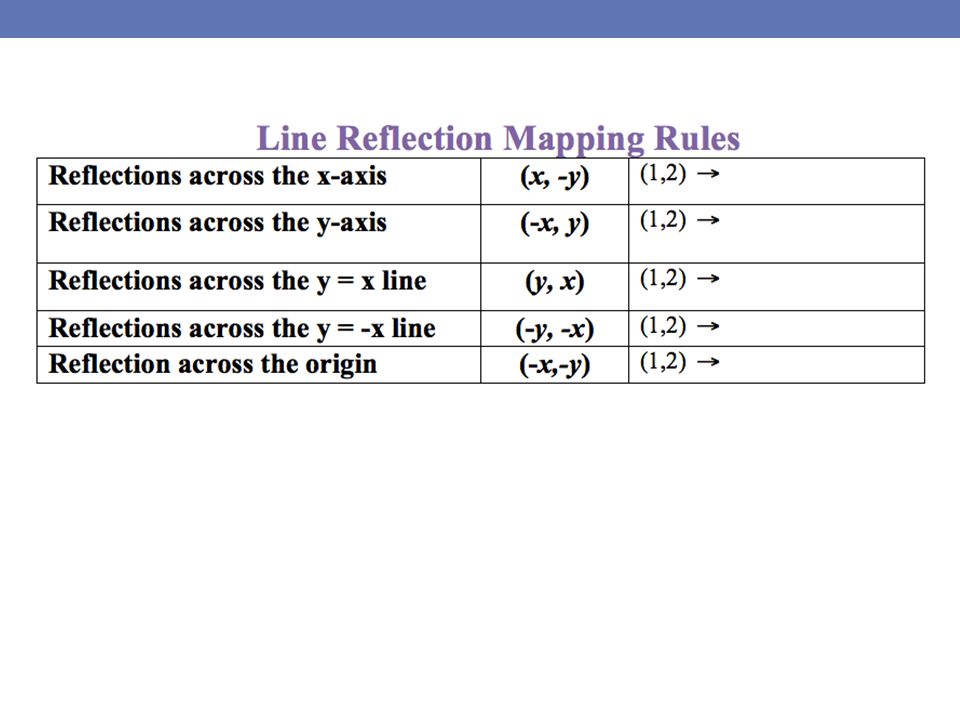
A reflection in a line produces a mirror image in which corresponding points on the original shape are always the same distance from the mirror line The reflected image has the same size as the original figure, but with a reverse orientation Examples of transformation geometry in the coordinate plane Reflection over y axis (x, y) ( x, y)Start studying Reflection Rules Learn vocabulary, terms, and more with flashcards, games, and other study toolsA reflection (or flip) is one kind of transformation The reflection of a point is another point on the other side of a line of symmetry Both the point and its reflection are the same distance from the line The following diagram show the coordinate rules for reflection over the xaxis, yaxis, the line y = x and the line y = x
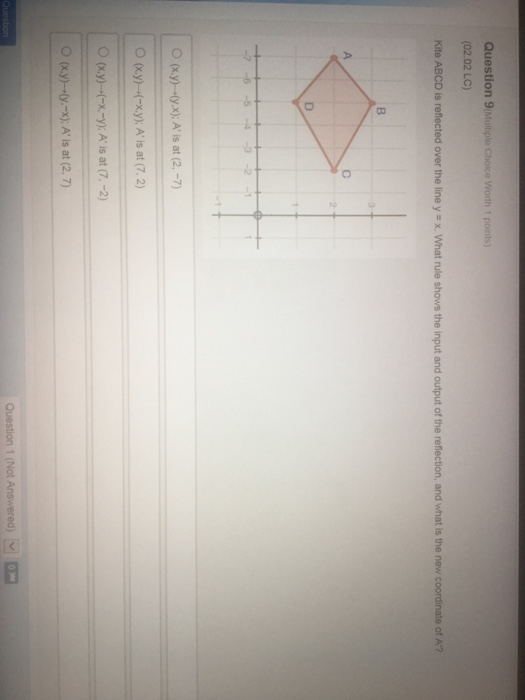
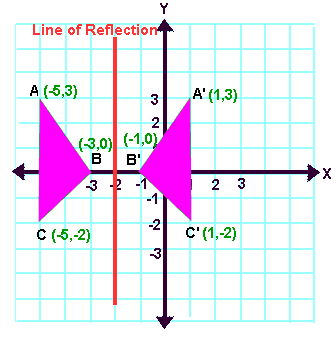
A shape can be reflected in the line y = −x If point on a shape is reflected in the line y = −x both coordinates change sign (the coordinate becomes negative if it is positive and vice versa) the xcoordinate becomes the ycoordinate and the ycoordinate becomes the xcoordinate The rule for reflecting over the X axis is to negate the value of the ycoordinate of each point, but leave the xvalue the same For example , when point P with coordinates (5,4) is reflecting across the X axis and mapped onto point P', the coordinates of P' are (5,4)Kite abcd is shown a is at negative 7, 2, b is at negative 5, 3, c is at negative 2, 2, d is at negative 5, 1
Apply a reflection over the line x=3 Since the line of reflection is no longer the xaxis or the yaxis, we cannot simply negate the x or yvalues This is a different form of the transformation Let's work with point A first Since it will be a horizontal reflection, where the reflection is over x=3, we first need to determine theA point reflection is just a type of reflection In standard reflections, we reflect over a line, like the yaxis or the xaxisFor a point reflection, we actually reflect over a specific point, usually that point is the origin $ \text{Formula} \\ r_{(origin)} \\ (a,b) \rightarrow ( \red a , \red b) $14 Reflections Over y = x, y = –x, y = #, & x = # Geometry Directions Write the rule of the transformation (This is a mixed review) 1) A line segment is reflected over y = –x 2) A line segment is translated 5 units left & 1 unit up




Reflection Rules How To W 25 Step By Step Examples



Question 14 Multiple Choice Worth 1 Points 02 02 Chegg Com
The rule for a reflection over the y axis is ( x , y ) → ( − x , y ) Reflection in the line y = x A reflection of a point over the line y = x is shownA (x, y)→(y, x);The resulting orientation of the two figures are opposite Corresponding parts of the figures are the same distance from the line of reflection Ordered pair rules reflect over the xaxis (x, y), yaxis (x, y), line y=x (y, x)




Reflection Transformation Matrix



Reflection Across The Y Axis With Rule Educreations
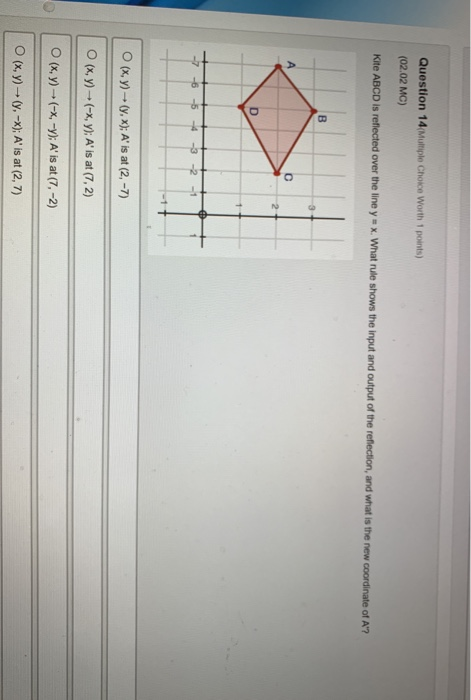
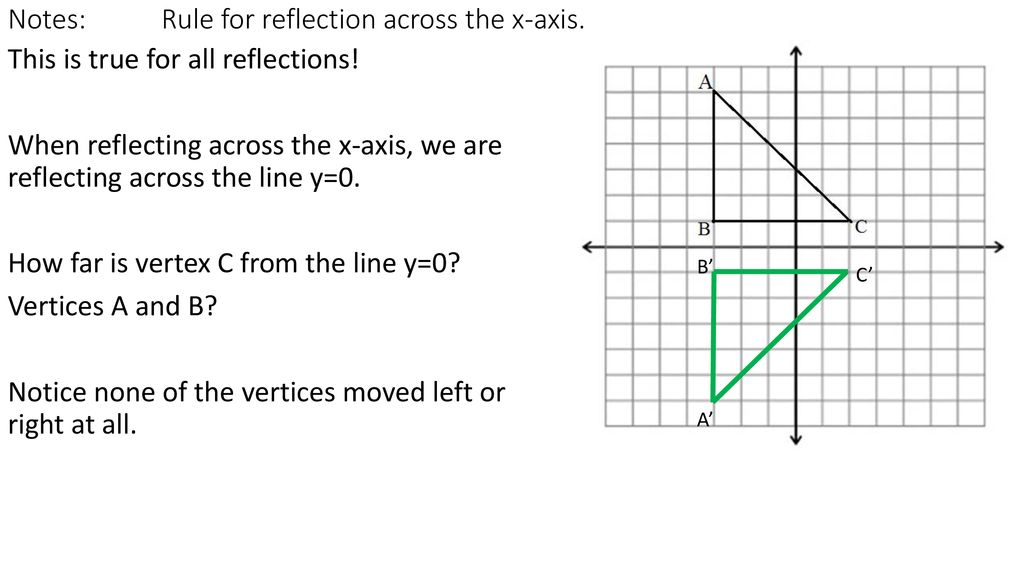
Correct answer to the question Kite abcd is reflected over the line y = x what rule shows the input and output of the reflection, and what is the new coordinate of a'?Just approach it stepbystep For each corner of the shape 1 Measure from the point to the mirror line (must hit the mirror line at a right angle) 2A reflection is defined by the axis of symmetry or mirror line In the above diagram, the mirror line is x = 3 Under reflection, the shape and size of an image is exactly the same as the original figure This type of transformation is called isometric transformation



Solved Question 9 Multiple Choice Worth 1 Points 02 02 Chegg Com




02 02 Trapezoid Abcd Is Reflected Over The Line Y X What Rule Shows The Input And Output Of The Brainly Com
Reflection Over y = 2 With Rule by Lance Powell on image/svgxml ShareQ What is the Algebraic Notation for this Reflection?Get the free "Reflection Calculator MyALevelMathsTutor" widget for your website, blog, Wordpress, Blogger, or iGoogle Find more Education widgets in WolframAlpha



Coordinate Rules For Reflections On A Graph Mov Youtube



Reflection Across The Diagonal With Rule Educreations
What point do you get if you reflect the point ( 6,1) over the line y = x?Over the yaxis (x, y) (–x, y) Over the line y = x (x, y) (y, x) Through the origin (x, y) (–x, –y) TRANSLATIONS Translations are a slide or shift Translations can be achieved by performing two composite reflections over parallel lines Translations are isometric, and preserve orientation Coordinate plane rules (x, y) (x ± h, y ±This lesson is presented by Glyn CaddellFor more lessons, quizzes and practice tests visit http//caddellpreponlinecomFollow Glyn on twitter http//twitter



Linear Reflections Across X And Y Axis Example Youtube



Reflections In Math Formula Examples Practice And Interactive Applet On Common Types Of Reflections Like X Axis Y Axis And Lines
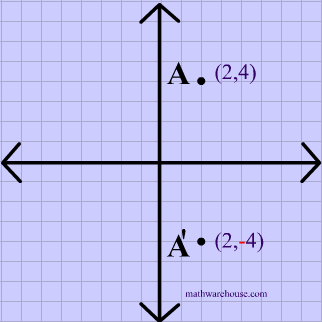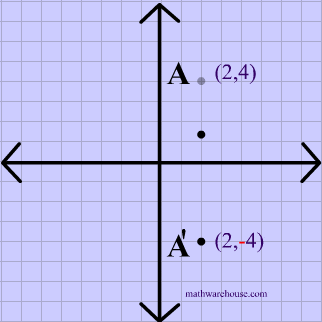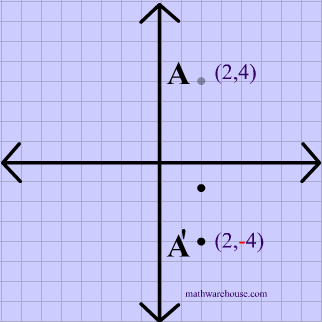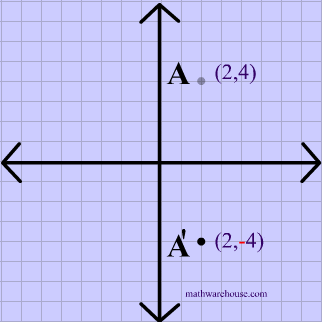
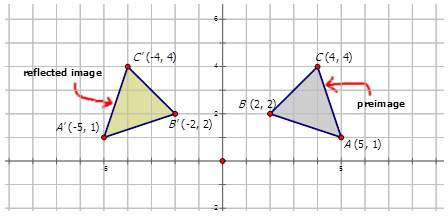
Q Reflect the point (2, 4) over the yaxis Q Point C (5, 4) is reflected over the xaxis What are the coordinates of C'?Reflection across the line y=x swaps the x and ycoordinates A(5, 1) becomes A'(1, 5), for example The coordinates of the other points are swapped in similar fashionWe're asked to use the reflection tool to define a reflection that will map line segments Emme line segments Emme on to the other line segments below so we want to map Emme to this segment over here and we want to use a reflection let's see let's see what they expect from us if we want to add a reflection so if I click on this it says reflection over the line from and then we have two



What Does It Mean To Reflect Over The Y X Line Quora




Reflection Over The Line Y X Geogebra
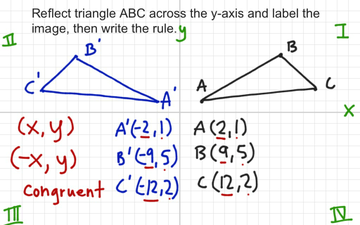
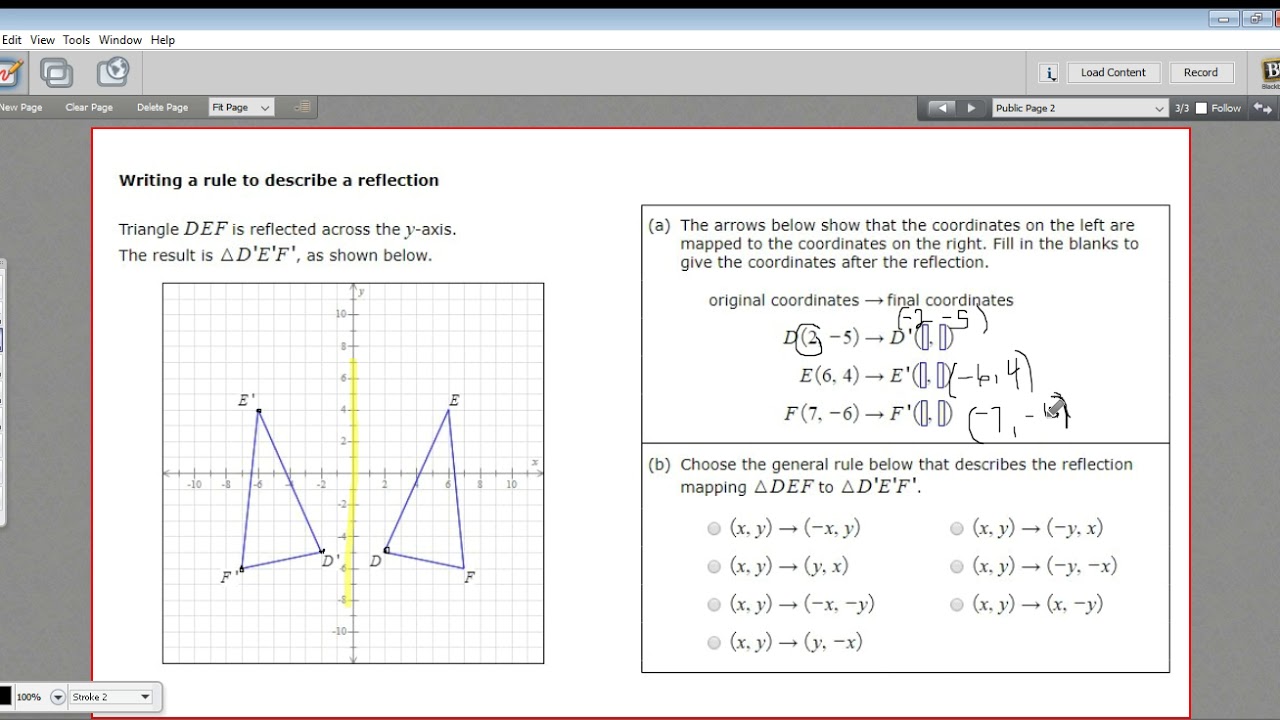
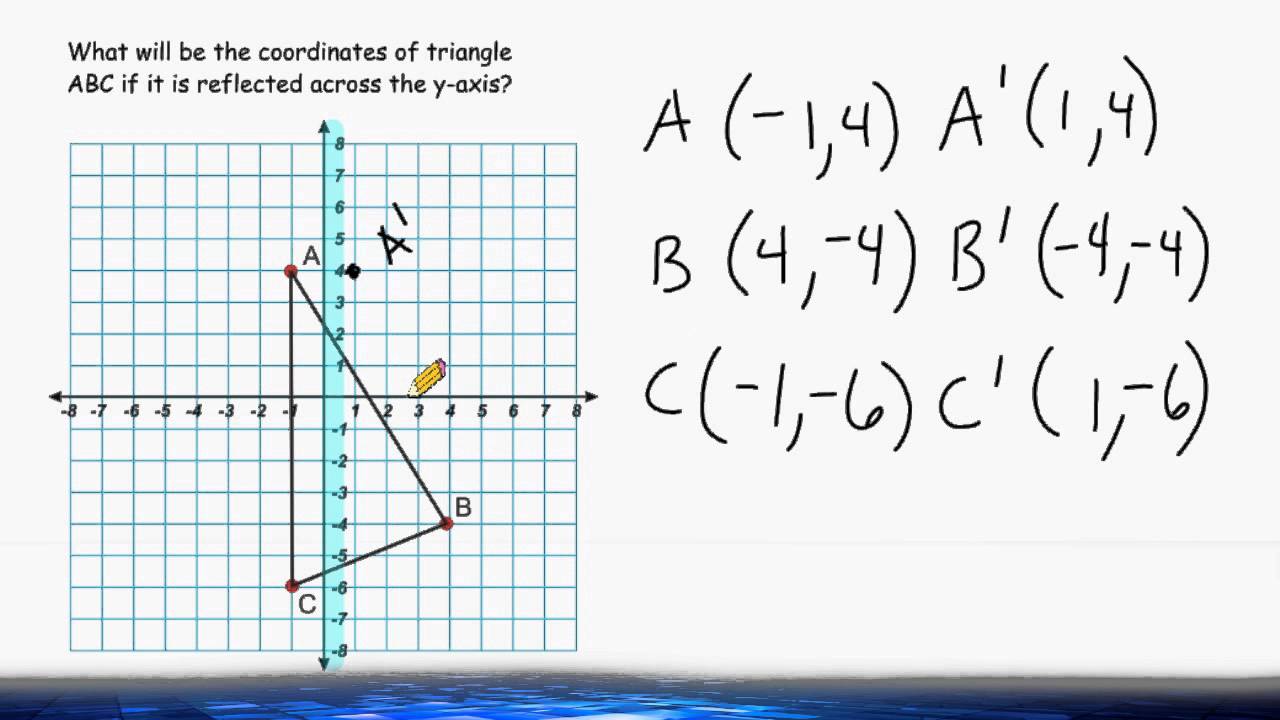
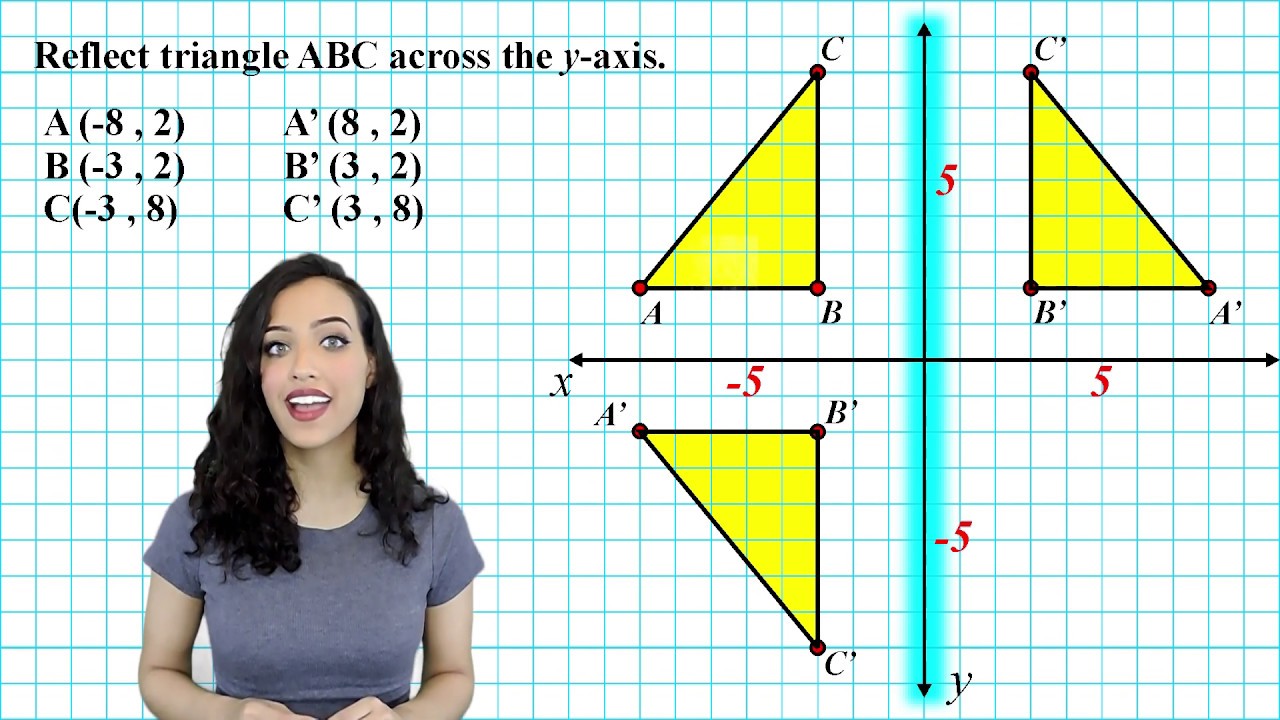
To perform a geometry reflection, a line of reflection is needed;Reflection in the line y = x Try to work out the coordinates of the reflected points before you reveal the answer 1) If the point (2, 3) is reflected over the xaxis what is the new point?Determine the line of reflection The most common lines of reflection are the xaxis, the yaxis, or the lines y = x or y=−x The preimage has been reflected across he yaxis This means, all of the xcoordinates have been multiplied by 1 You can describe the reflection in words, or with the following notation ry−axis(x,y)→(−x,y)




D Reflection Across Y X Brainly Com



1
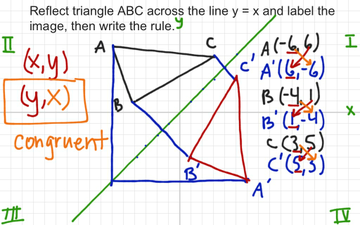
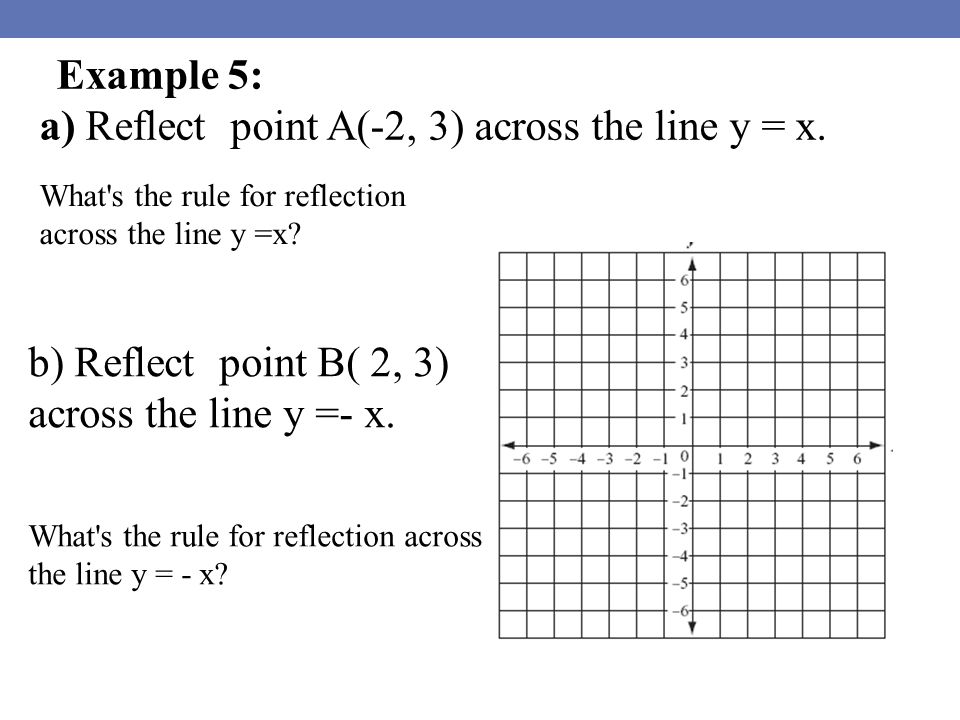
Y=x reflection rule A 33 B 21 and C 62 would turn into A 33 B 12 and C 26 Reflection Over Y X In order to define or describe a reflection you need the equation of the line of reflection For triangle ABC with coordinate points A 33 B 21 and C 62 apply a reflection over the line yx Reflection Over y 2 With Rule by Lance Powell on Reflection in a Line 4 Reflecting over the line y = x or y = x (the lines y = x or y = x as the lines of reflection) When you reflect a point across the line y = x, the xcoordinate and the ycoordinate change placesReflection Rule over the line y = x Get the answers you need, now!




Quiz Worksheet Graphing The Origin Y X Reflections Across Axes Study Com




View Question If A Reflection In The Line Y X Occurs Then The Rule For This Reflection Is
Q The point ( 2,5) is reflected over the lineKite abcd is reflected over the line y = x what rule shows the input and output of the reflection, and what is the new coordinate of a'?When you reflect a point across the line y = x, the xcoordinate and the ycoordinate change places or Reflecting over any other line Notice how each point of the original figure and its image are the same distance away from the line of reflection (x = –2 in this example) What is the rule for a reflection over Y = X?



What Does It Mean To Reflect Over The Y X Line Quora
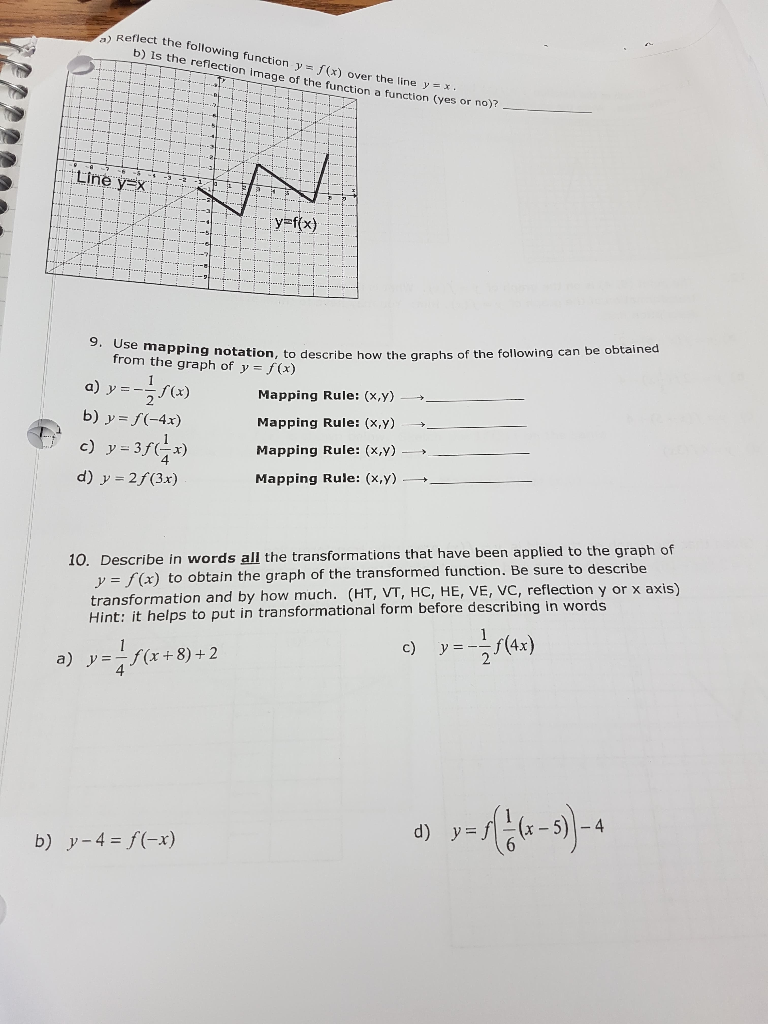



Reflect The Following Function Y F X Over The Line Chegg Com
Problem 1 Find a linear transformation rule of the form (p, q) → (r, s) such that the reflection image of the point (p, q) over the oblique line y = mx b is the point (r, s) In the general case, both r and s are functions of p, q, m and bA' is at (2, −7) b (x, y)→(−x, y);Play this game to review Geometry B(2, 4) Reflect over the line y = xNov 04, 15 Write a rule in function notation to describe the transformation that is a reflection across the yaxis A Rx0(X,Y) B Ry0(X,Y) C Ryx(X,Y) D Rx1(X,Y) math Triangle ABC below is reflected across the yaxis and then translated 1 unit right and 2 units downThe incident ray, the reflected ray, and the normal to the




Learn About Reflection Over The Line Y X Caddell Prep Online
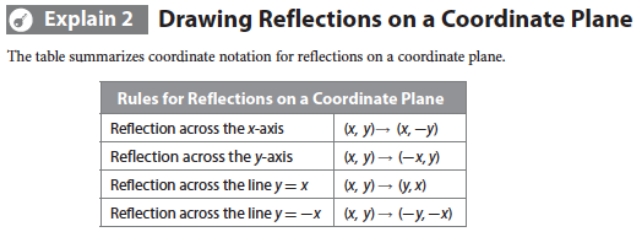



Reflections Day 1 Geogebra
A reflection is a flip over a line You can try reflecting some shapes about different mirror lines here How Do I Do It Myself?A' is at (7, 2) c hmwhelpercom🔴 Answer 1 🔴 on a question Kite abcd is reflected over the line y = x what rule shows the input and output of the reflection, and what is the new coordinate of a'?




Answered Rules For Reflections On A Coordinate Bartleby
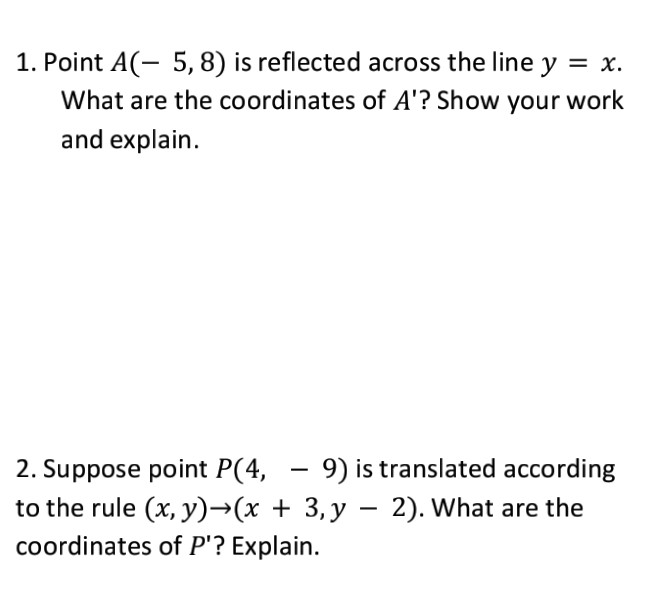



1 Point A 5 8 Is Reflected Across The Line Y X Chegg Com
90˚ counterclockwise rotation about the origin OR 270˚ clockwise rotation about the originNote the rule of reflection over the line y = x is (x,y) > (x,y) according to this rule, each x and y coordinates became opposite in signs of the original given coordinate (x,y) in the given options, we have option d using same rule (x,y)→(−x,−y) therefore, correct option would be d optionTrapezoid abcd is reflected over the line y = x what rule shows the input and output of the reflection, and what is the new coordinate of a'?




Reflection Mathbitsnotebook A1 Ccss Math
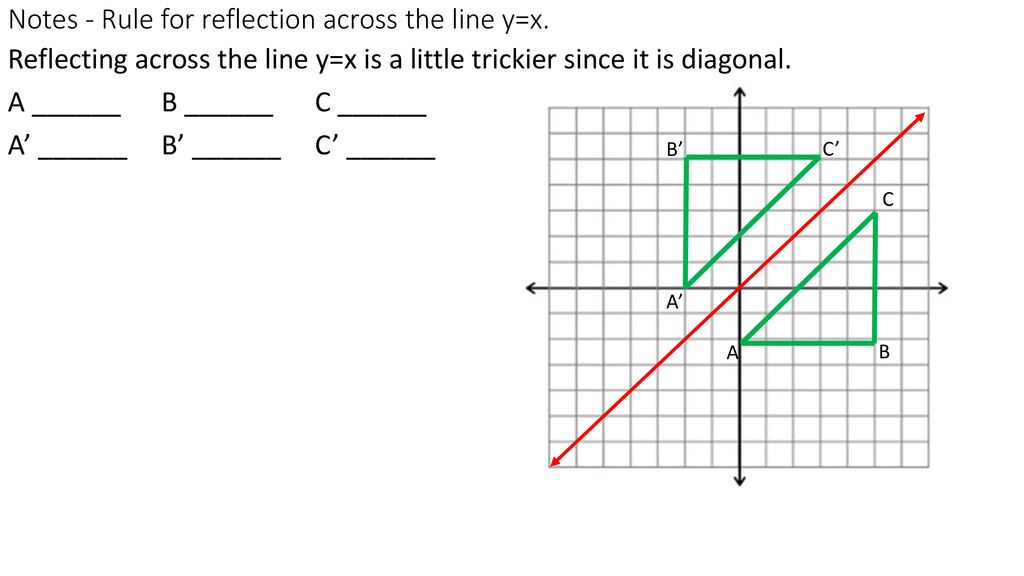



Unit 6 Day Ppt Download
Q Which rule describes the action of the coordinates when a figure is reflected over the line y = x? We have to identify the rules of reflection Firstly, the rule for reflecting a point about the line y=x is While reflecting about the line y=x, we get the reflected points by swapping the coordinates So, Option 3 is correctThe linear transformation rule (p, s) → (r, s) for reflecting a figure over the oblique line y = mx b where r and s are functions of p, q, b, and θ = Tan 1 (m) is shown below Finding the linear transformation rule given the equation of the line of reflection equation y = mx b involves using a calculator to find angle θ = Tan 1 (m




Reflecting Shapes Article Reflections Khan Academy




Reflection Over Y 2 With Rule Educreations
Reflections across y=x Click and drag the blue dot and watch it's reflection across the line y=x (the green dot) Pay attention to the coordinatesTo reflect a figure across a line of reflection, reflect each of its vertices Then connect the vertices to form the image Each point and its image must be at the same distance from the line of reflection We can use the rules shown in the table for changing the signs of the coordinates after a reflectionKite ABCD is reflected over the line y = x What rule shows the input and output of the reflection, and what is the new coordina



Reflection Rules Toolkit




Rules For Reflections Read Geometry Ck 12 Foundation
When reflecting coordinate points of the preimage over the line, the following notation can be used to determine the coordinate points of the image r y=x =(y,x) For example For triangle ABC with coordinate points A(3,3), B(2,1), and C(6,2), apply a reflection over the line y=x By following the notation, we would swap the xvalue and the yvalue When reflecting over the line y=x, we switch our x and y, and make both negative Reflection Over Y = X In order to define or describe a reflection, you need the The notation or rule for a reflection over the line y=x (x, y) → (y, x)



What Does It Mean To Reflect Over The Y X Line Quora




Transformations Of Graphs
1 Log in Join now 1 Log in Join now Middle School Mathematics 5 points Keylonparker35 Asked Reflection Rule over the line y = x See answers (1) Ask for details ;A reflection in the line y = x can be seen in the picture below in which A is reflected to its image A' The general rule for a reflection in the y = x (A, B) → (B, A)Follow Report Log in to add a




Reflections In Math Formula Examples Practice And Interactive Applet On Common Types Of Reflections Like X Axis Y Axis And Lines




Answered Rules For Reflections On A Coordinate Bartleby
VIEW ENTIRE PLAYLIST http//wwwyoutubecom/view_play_list?p=0D2E061AF3327C If this helps, please leave a comment and subscribe!2) If the point (4, 6) is reflected over the yaxis what is the new point?Reflection about the line y=x Once students understand the rules which they have to apply for reflection transformation, they can easily make reflection transformation of a figure For example, if we are going to make reflection transformation of the point (2,3) about xaxis, after transformation, the point would be (2,3)




Reflection Over The X And Y Axis The Complete Guide Mashup Math




Reflections In Math Formula Examples Practice And Interactive Applet On Common Types Of Reflections Like X Axis Y Axis And Lines




Reflections In Math Formula Examples Practice And Interactive Applet On Common Types Of Reflections Like X Axis Y Axis And Lines



Reflection An Isometry Or Rigid Motion In Which A Figure Is Flipped Giving Its Image An Opposite Orientation Ppt Download



Unit 6 Day Ppt Download




Reflection Mathbitsnotebook A1 Ccss Math




Reflecting Figures In Coordinate Space Krista King Math Online Math Tutor




Reflection Rules How To W 25 Step By Step Examples




Write The Rule For A Reflection Over The Line Y X 2 Picture Down Below Pls Help And Show Work Brainly Com




Reflection Over The Line Y X Youtube
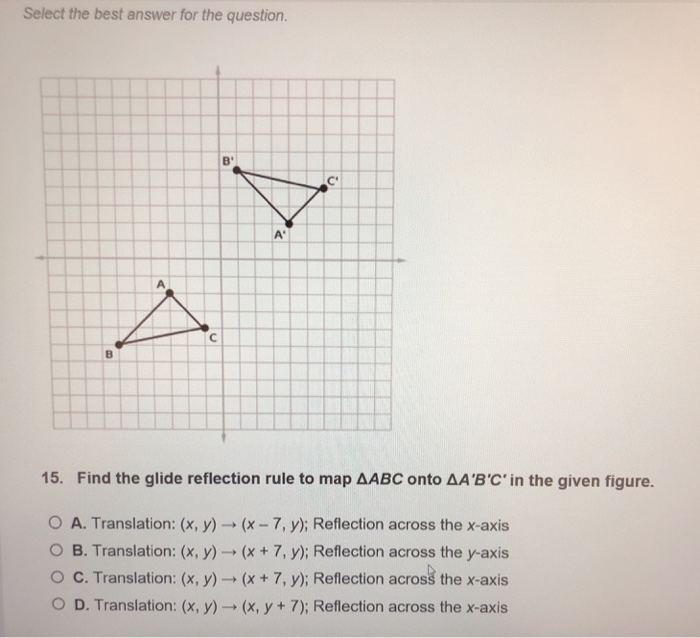



Select The Best Answer For The Question 15 Find The Chegg Com



60 Intro To Dilations 5 James Parson Library Formative



1



Rules For Reflections Read Geometry Ck 12 Foundation
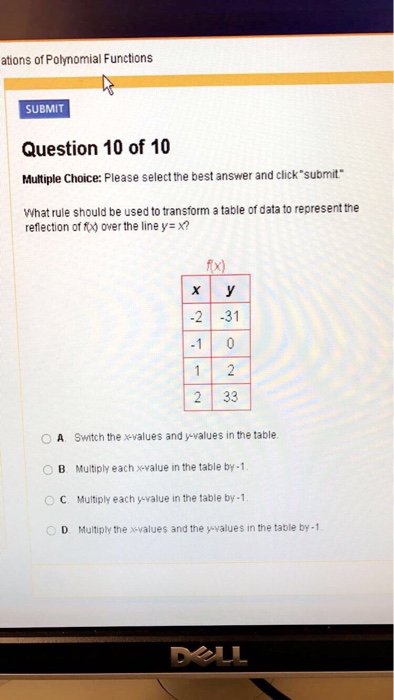



What Rule Should Be Used To Transform A Table Of Data Chegg Com



Www Pmschools Org Site Handlers Filedownload Ashx Moduleinstanceid 701 Dataid 3614 Filename 15 composition of transformations answers Pdf




Reflection Over Y X Math Geometry Showme
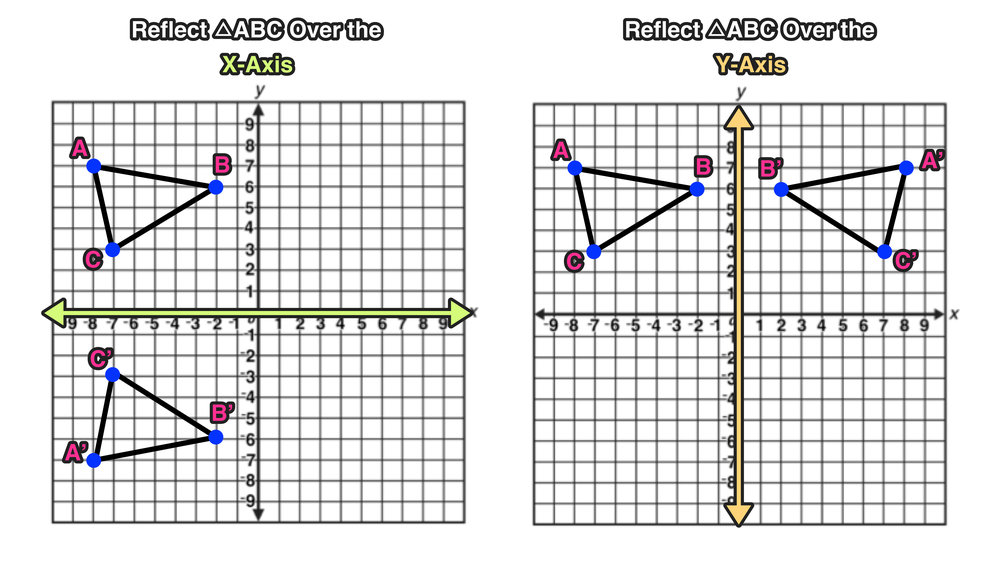



Reflection Over The X And Y Axis The Complete Guide Mashup Math




Transformation Reflection Over The Line Y X Youtube




A What Are The Coordinates Of The Image Of Vertex G After A Reflection Across The Line Y X Brainly Com




Kite Abcd Is Reflected Over The Line Y X What Rule Shows The Input And Output Of The Reflection Brainly Com




Rectangle Abcd Is Reflected Over The X Axis What Rule Shows The Input And Output Of The Reflection Brainly Com



Reflection Transformation Matrix



Reflection Rules How To W 25 Step By Step Examples




Reflection Mathbitsnotebook A1 Ccss Math




Reflection Over The Line Y X Math Showme




Reflection Over The X And Y Axis The Complete Guide Mashup Math




Reflection In Geometry Examples Solutions Videos Worksheets Games Activities




Ppt Reflect Over Y X Powerpoint Presentation Free Download Id




Reflection Definition Reflection In The Coordinate Plane




Reflections Through The Axes And The Lines Y X And Y X Geogebra




Reflections Rules Review For Transformation Test Youtube



Www Pmschools Org Site Handlers Filedownload Ashx Moduleinstanceid 701 Dataid 3614 Filename 15 composition of transformations answers Pdf




Rules For Reflections Read Geometry Ck 12 Foundation




Reflection Over The Y X Line Youtube




Geometry Transformations



Www Pmschools Org Site Handlers Filedownload Ashx Moduleinstanceid 701 Dataid 3614 Filename 15 composition of transformations answers Pdf



Writing A Rule To Describe A Reflection Youtube
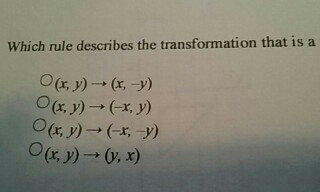



Which Rule Describes The Transformation That Is A Chegg Com
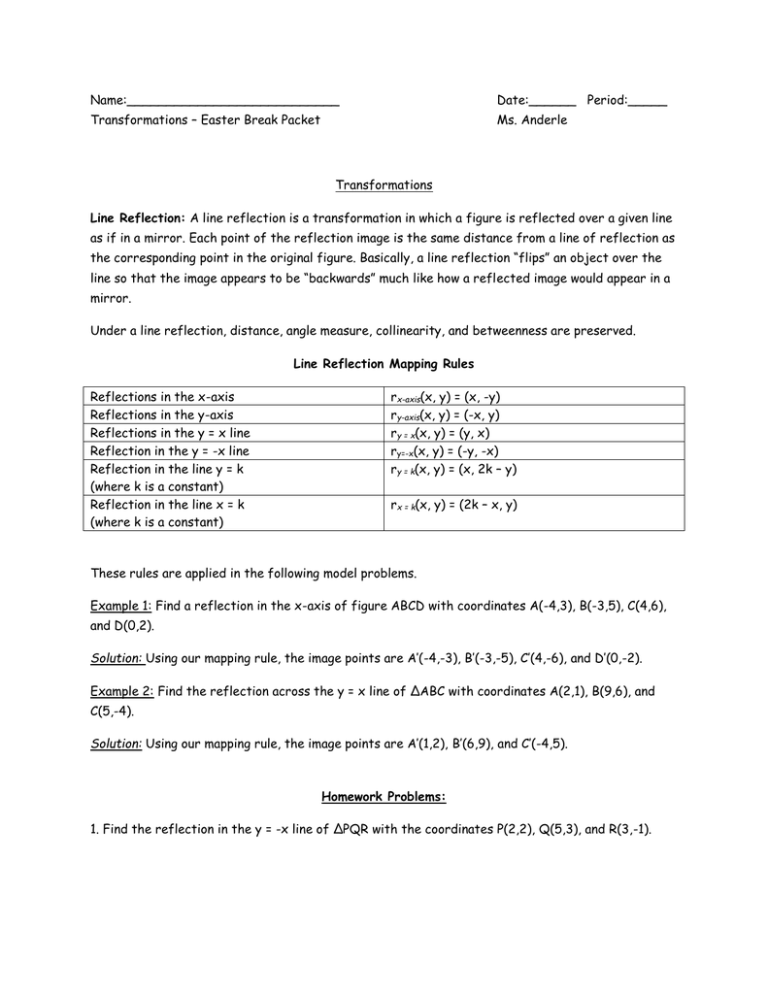



Name Date Period Transformations Easter Break Packet




Geometry Identify The Reflection Rule On A Coordinate Plane That Verifies That Triangle A 1 7 Brainly Com



Reflection An Isometry Or Rigid Motion In Which A Figure Is Flipped Giving Its Image An Opposite Orientation Ppt Download



1




Reflections




Reflection Transformation Matrix




A Line Segment Goes From 1 2 To 4 1 The Line Segment Is Reflected Across X 1 Reflected Across Y 3 And Then Dilated About 2 2 By A Factor




Reflection In The Line Y X Geogebra



Reflection Across The X And Y Axis Youtube



Reflection Across The X And Y Axis Youtube




Transformations Boundless Algebra



Reflection Of A Point In A Line Assignment Point
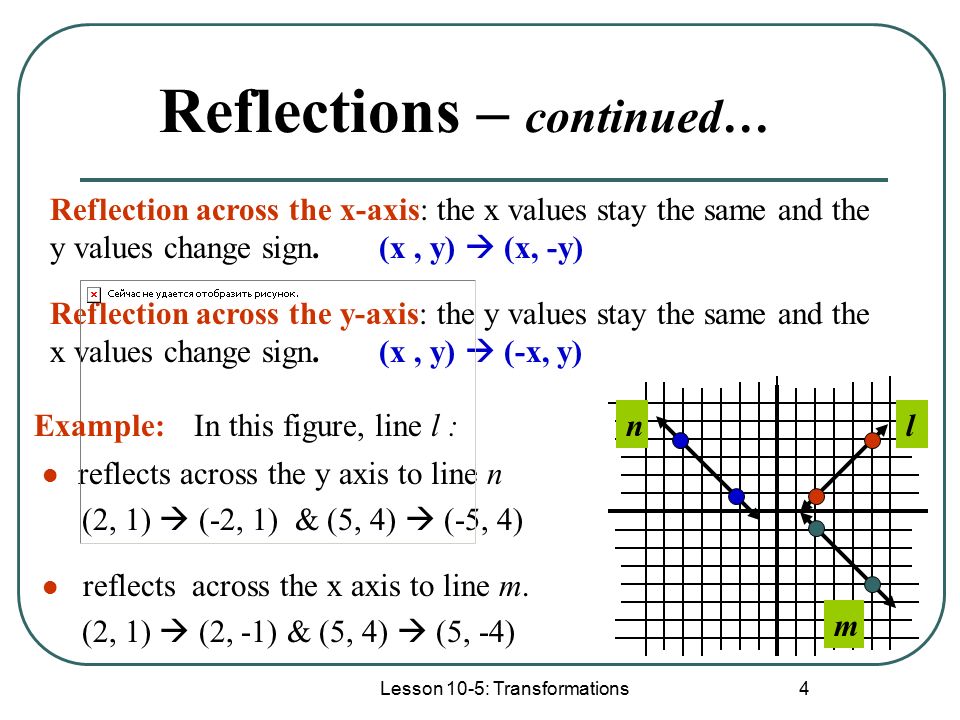



Lesson 10 5 Transformations 1 Lesson 10 5 Transformations Ppt Download



Schoolwires Henry K12 Ga Us Cms Lib08 Ga Centricity Domain 26 7th and 8th grade math 8th grade flexbook Unit 1 sections 1 23 1 4 rules for reflections Pdf
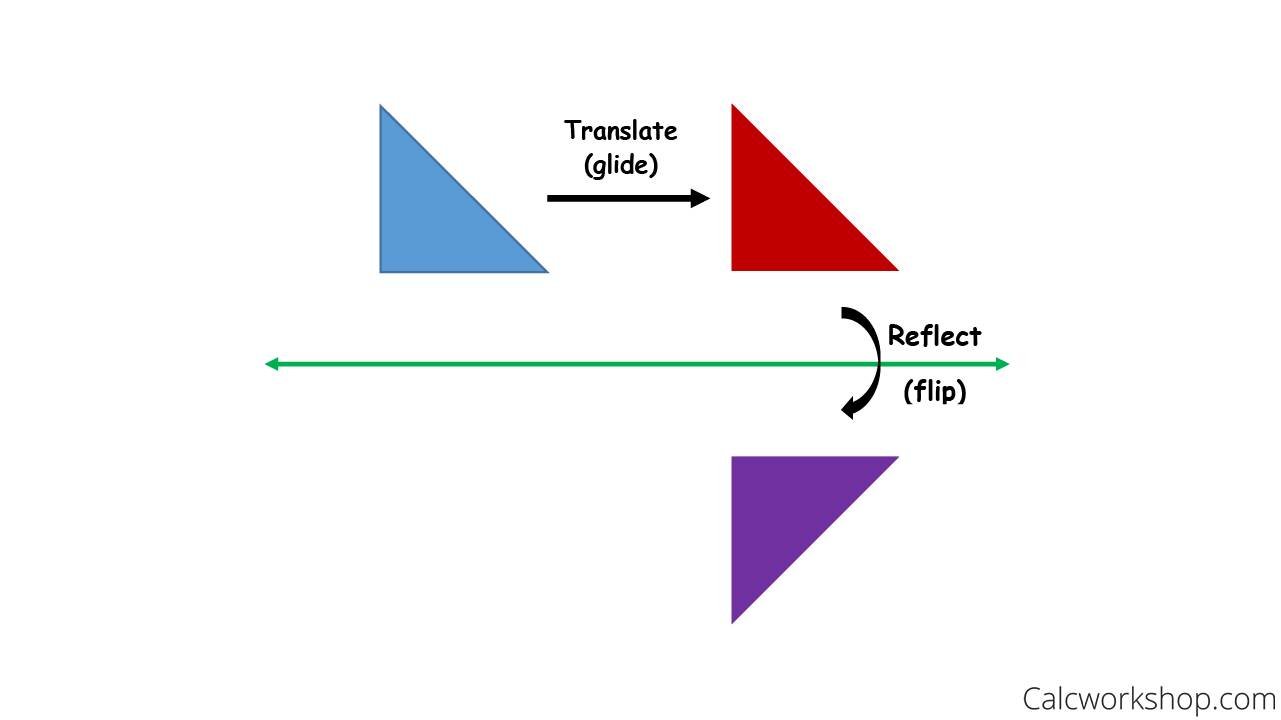



Reflection Rules How To W 25 Step By Step Examples



Schoolwires Henry K12 Ga Us Cms Lib08 Ga Centricity Domain 26 7th and 8th grade math 8th grade flexbook Unit 1 sections 1 23 1 4 rules for reflections Pdf




9 2 Reflections Key Concepts R Stands For Reflection And The Subscript Tells You What To Reflect On Ex R X Axis The Line Of Reflection Is What Ppt Download
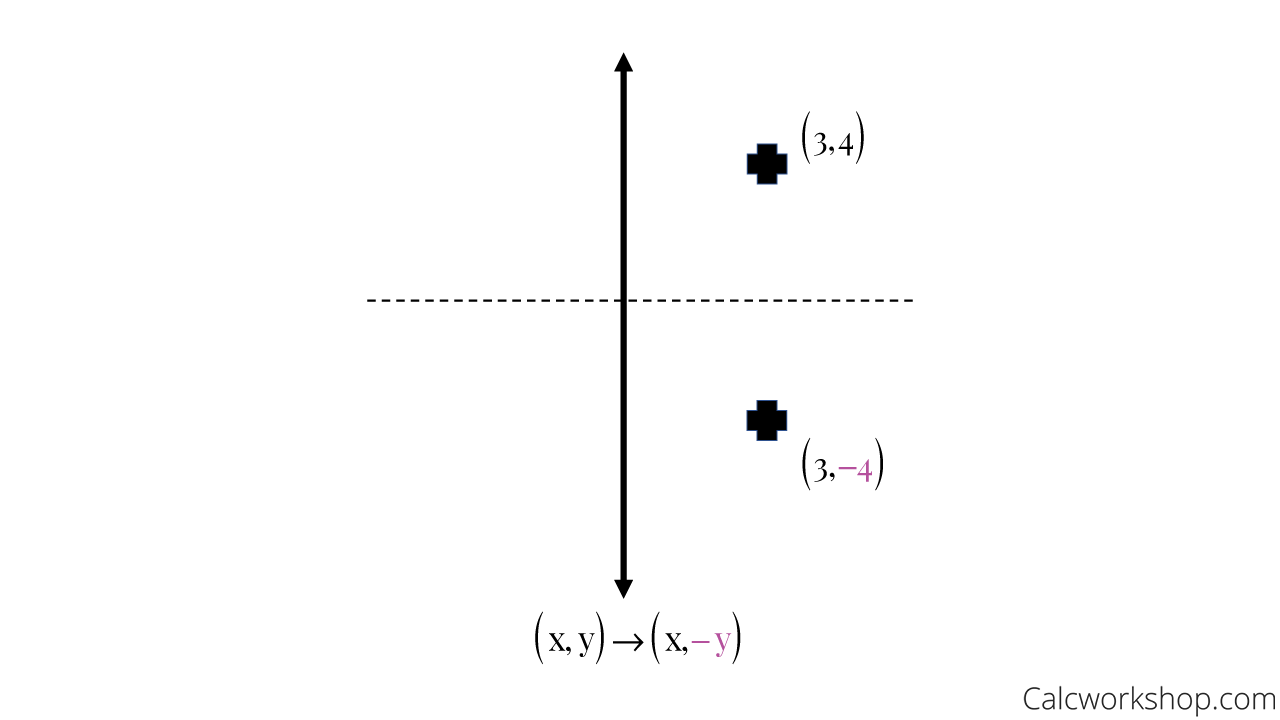



Reflection Rules How To W 25 Step By Step Examples



Www Cabarrus K12 Nc Us Cms Lib Nc Centricity Domain 4633 U1 review answers Pdf



1
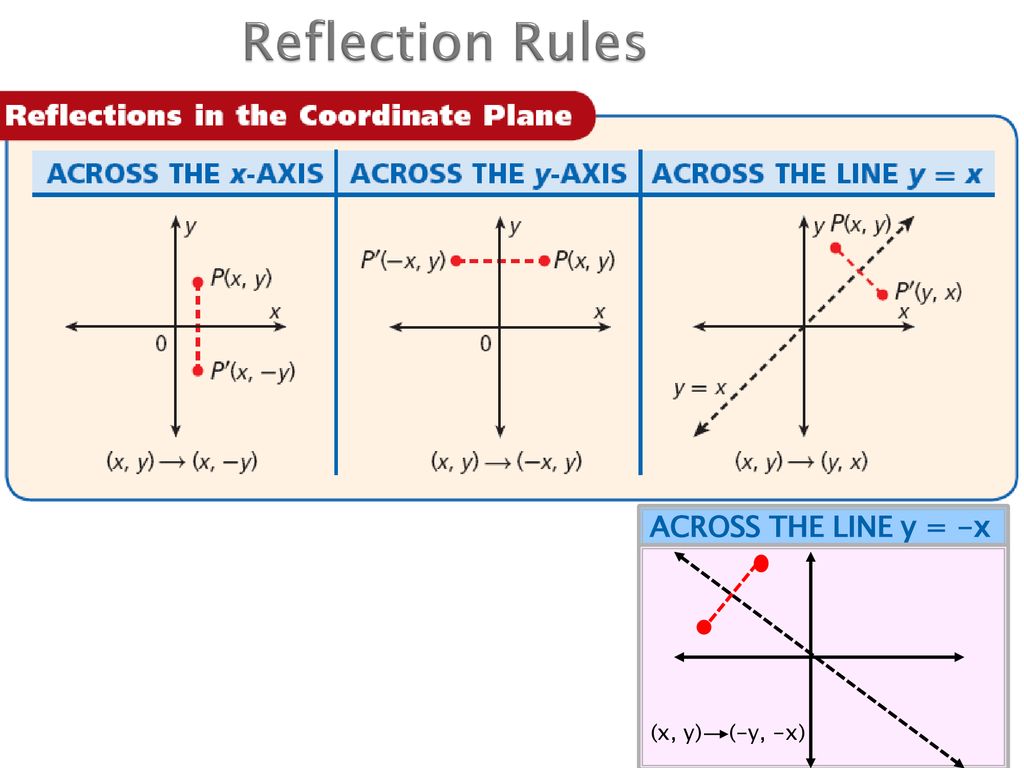



Pearson Unit 2 Topic 8 Transformational Geometry 8 2 Reflections Pearson Texas Geometry C 16 Holt Geometry Texas C Ppt Download
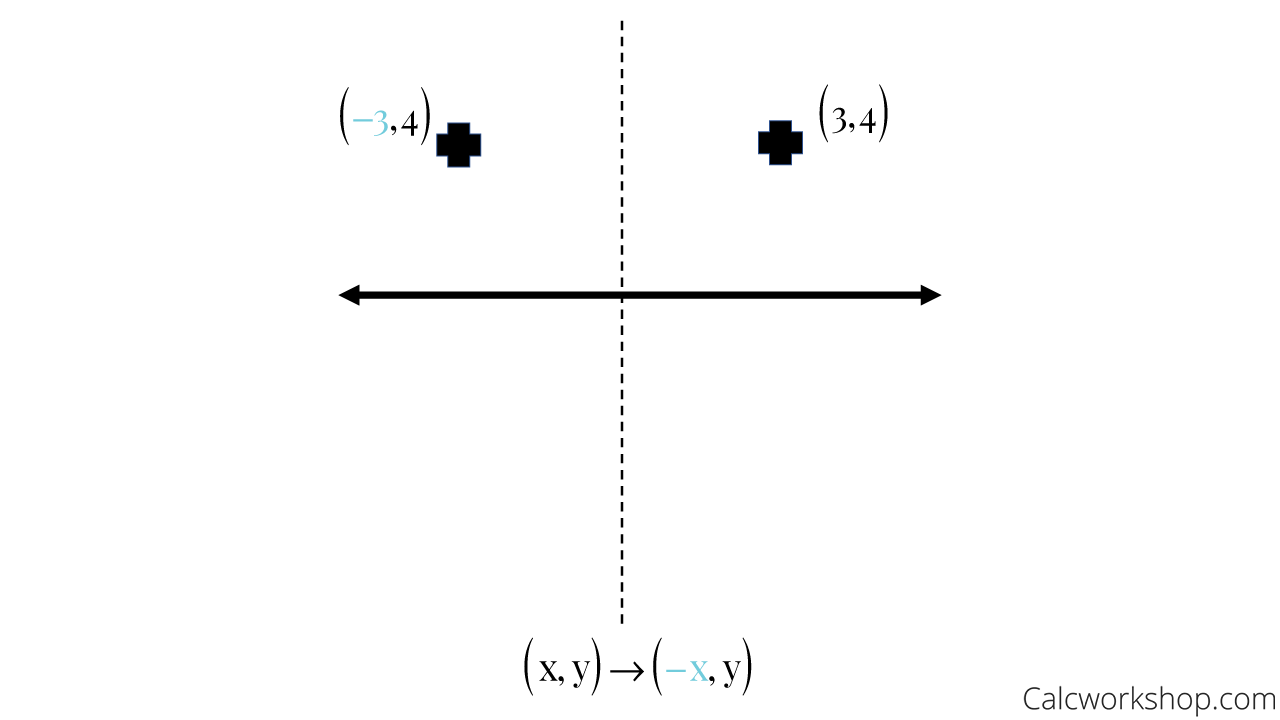



Reflection Rules How To W 25 Step By Step Examples




How To Graph Reflections Across Axes The Origin And Line Y X Video Lesson Transcript Study Com



Ch 3 4
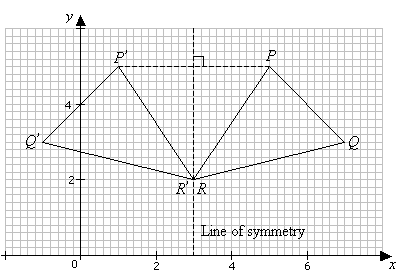



Reflection Transformation Solutions Examples Videos




Reflection Mathbitsnotebook A1 Ccss Math




Trapezoid Abcd Is Reflected Over The Line Y X What Rule Shows The Input And Output Of The Brainly Com




Date Period 17 Which Of The Following Describes The Chegg Com




Write A Rule To Describe Each Transformation What Is The Reflection Ex Reflect With Reaparece To Brainly Com




Reflection Transformation Matrix




Reflection Rules How To W 25 Step By Step Examples




Algebraic Representations Of Reflections




Reflection Mathbitsnotebook A1 Ccss Math



0 件のコメント:
コメントを投稿